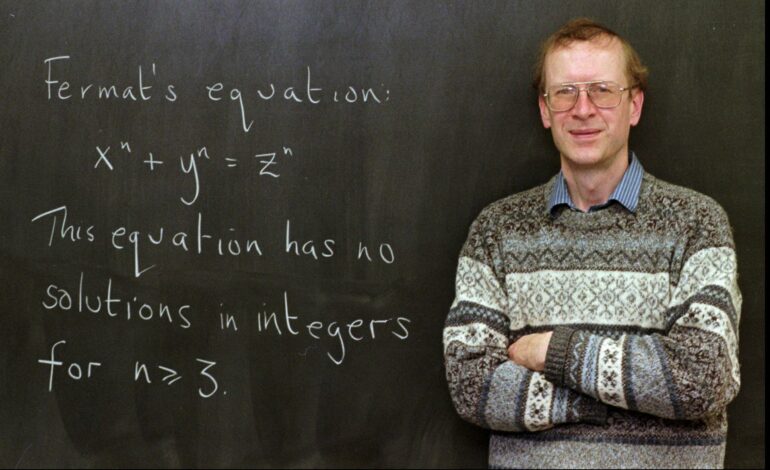
On June 23, 1993, the mathematician Andrew Wiles gave the last of three lectures detailing his solution to Fermat’s last theorem, a problem that had remained unsolved for three and a half centuries. Wiles’ announcement caused a sensation, both within the mathematical community and in the media.
Beyond providing a satisfying resolution to a long-standing problem, Wiles’ work marks an important moment in the establishment of a bridge between two important, but seemingly very different, areas of mathematics.
History demonstrates that many of the greatest breakthroughs in math involve making connections between seemingly disparate branches of the subject. These bridges allow mathematicians, like the two of us, to transport problems from one branch to another and gain access to new tools, techniques and insights.
What is Fermat’s last theorem?
Fermat’s last theorem is similar to the Pythagorean theorem, which states that the sides of any right triangle give a solution to the equation x2 + y2 = z2 .

The Pythagorean theorem, named for the Ancient Greek philosopher Pythagorus, is a fundamental result in Euclidean geometry that relates the lengths of the sides of a right triangle.
AmericanXplorer13 via Wikimedia Commons, CC BY-SA 3.0
Every differently sized triangle gives a different solution, and in fact there are infinitely many solutions where all three of x, y and z are whole numbers – the smallest example is x=3, y=4 and z=5.
Fermat’s last theorem is about what happens if the exponent changes to something greater than 2. Are there whole-number solutions to x3 + y3 = z3 ? What if the exponent is 10, or 50, or 30 million? Or, most generally, what about any positive number bigger than 2?

The French mathematician Pierre de Fermat.
Rolland Lefebvre via Wikimedia Commons
Around the year 1637, Pierre de Fermat claimed that the answer was no, there are no three positive whole numbers that are a solution to xn + yn = zn for any n bigger than 2. The French mathematician scribbled this claim into the margins of his copy of a math textbook from ancient Greece, declaring that he had a marvelous proof that the margin was “too narrow to contain.”
Fermat’s purported proof was never found, and his “last theorem” from the margins, published posthumously by his son, went on to plague mathematicians for centuries.
Searching for a solution
For the next 356 years, no one could find Fermat’s missing proof, but no one could prove him wrong either – not even Homer Simpson. The theorem quickly gained a reputation for being incredibly difficult or even impossible to prove, with thousands of incorrect proofs put forward. The theorem even earned a spot in the Guinness World Records as the “most difficult math problem.”
That is not to say that there was no progress. Fermat himself had proved it for n=3 and n=4. Many other mathematicians, including…