Optical fibres made of topological photonic crystals allow improved versatility and control across the modes and polarization of light they transmit. Compositionally, photonic crystals contain bandgaps to prevent the passage of light relative to specific wave energies and momenta much like an on/off switch. In a new report now published on Nature Light: Science & Applications, Hao Lin, and Ling Lu at the Institute of Physics at the Chinese Academy of Sciences transmitted pure “single mode” light across a large frequency range via a topological feature known as a “Dirac vortex.” The concept can lead to applications that transmit light signals more stably across long distances. While the work is theoretical at present, the researchers suggest the use of fibers made from silica based on stack-and-draw methods or three-dimensional (3-D) printing technologies to fabricate and test these theoretical concepts.
Understanding the nodal lines and Weyl points in a photonic crystal fibre.
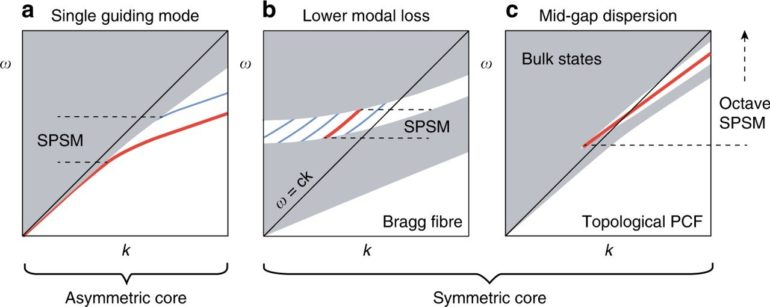
Photonic crystal fibres rely on the endless variety of two-dimensional (2-D) crystals for their functionality. The concept of topological photonics based on robust waveguides can inspire new fibre concepts including the development of a one-way fibre inside a magnetic, 3-D photonic crystal. In this work, Lin and Lu introduced a topological photonic crystal fibre (PCF) resembling the Dirac vortex topological cavity in its cross-section using 2-D photonic crystals. The Dirac vortex fibre is an ideal design to develop ultrabroadband single-polarization single-mode (SPSM) fibres due to the singlet mid-gap dispersion within the bandgap. The scientists eased the fabrication step by introducing a simplified design of only four capillary silica tubes to finally achieve an octave-spanning SPSM.
The team began with the most common photonic crystal fibre, a silica photonic crystal with a triangular lattice of air holes. The material contained two nodal lines of 2-D Dirac points in the Brillouin zone. If they broke the inversion symmetry of the photonic crystal fibre by adding an extra small air hole into the primitive cell, each nodal line of the construct could gap into Weyl points or topological charges on the material. Weyl particles are elusive fermionic particles with vanishing mass and are not found as an elementary particle in nature. They are found instead to emerge in solid-state materials where 3-D bands can develop a topologically protected point-like crossing, known as a Weyl point. Photonic Weyl points can be realized in 3-D photonic crystals with complex structures.
Developing a generalised Kekulé structure
The topological bound state examined in this work can also be realized in honeycomb lattices with alternating single and double-bond representations of organic compounds known as Kekulé structures, named after the German chemist August Kekulé, who originally proposed the representation to develop the benzene organic compound. The researchers next coupled the two nodal lines of Dirac points in an enlarged supercell and annihilated them into a bandgap. Each supercell had three primitive cells labelled as an artificial ‘atom’ containing three struts. They moved each atom in the structure by shifting its centre of mass in any direction by adjusting the thickness of the three struts without changing the total mass of the atom. The scientists developed Kekulé modulations of the lattices and plotted their corresponding band structure.
The stability of the Dirac vortex photonic crystal fibre remained in the design itself since the corresponding topological defect was not formed by adding or removing material locally. The topological defect was formed by gently perturbing the whole lattice to create small local imperfections. A defining topological feature of the Dirac vortex fibre was the ease of creating multiple near degenerate modes by increasing the winding number – i.e. an integer representing the number of times that curves travel counterclockwise around a point, of the vortex. In principle, the researchers can practically fabricate a continuous, single-mode or multimode Dirac vortex photonic crystal fibre either from 3-D printed preforms (a form or shape), or via the stack-and-draw method used to develop optical fibres with more than a hundred tubes with different tube thicknesses. However, neither of these methods were convenient, therefore Lin and Lu et al. presented a discrete version of fibre design.
Fibre design with four tubes to form single-polarization single-mode fibres
The researchers only required four tubes to stack and draw the Dirac vortex photonic crystal fibre, which was very reasonable for fabrication. The four silica tubes used in the process had the same outer diameter to maintain the lattice, but different inner diameters for modulation. The researchers plotted the resulting discrete Dirac vortex fibre and its bound structure in which structural nonuniformity was present at six identical interfaces. They also noted the presence of index-guided modes within the Dirac vortex fibre that occurred wherever a sharp local maximum of the strut thickness was present. These were equivalent to a local rise of the effective refractive index. The scientists also noted the fibre cross-section with the vortex size and the corresponding band structure.
The Dirac vortex photonic crystal fibre with a finite vortex diameter maintained a single-polarization single-mode (SPSM). Lin and Lu et al. tested their potential performance relative to the confinement loss, dispersion and effective area and bending loss. They plotted the modes with the lowest confinement loss and noted the loss of the topological mode to be the lowest for the whole wavelength range across one octave . The specifications of the Dirac vortex photonic crystal fibre detailed here were similar to those in previous studies, although with the key difference of a single-polarization mode, used in this work.
Outlook for photonic crystal fibres
In this way, Hao Lin and Ling Lu numerically investigated the Dirac vortex topological photonic crystal fibre relative to its principle, construction and potential performance. They proposed developing this fibre using the standard stack-and-draw process using silica glass tubes or 3-D printed preforms. The method is advantageous compared to previous fibres due to its ability to guide any number of near-degenerate modes at will. The single-mode design provided the single-polarization, single-mode fibre with an octave bandwidth to easily tune the effective mode area by changing the vortex size in the material. The work suggests using photonic crystal fibres as a new platform for topological photonics.
Symmetry-enforced three-dimension Dirac phononic crystals
More information:
Lin H. & Lu L. Dirac-vortex topological photonic crystal fibre, Light: Science & Applications, doi.org/10.1038/s41377-020-00432-2
Lu L. et al. Topological photonics, Nature Photonics, doi.org/10.1038/nphoton.2014.248
Gao X. et al. Dirac-vortex topological cavities. Nature Nanotechnology doi.org/10.1038/s41565-020-0773-7
2020 Science X Network
Citation:
Designing Dirac vortex topological photonic crystal fibres (2020, December 30)
retrieved 31 December 2020
from https://phys.org/news/2020-12-dirac-vortex-topological-photonic-crystal.html
This document is subject to copyright. Apart from any fair dealing for the purpose of private study or research, no
part may be reproduced without the written permission. The content is provided for information purposes only.
