By cleverly applying a computational technique, scientists have made a breakthrough in understanding the “pseudogap,” a long-standing puzzle in quantum physics with close ties to superconductivity. The discovery, presented in Science, will help scientists in their quest for room-temperature superconductivity, a holy grail of condensed matter physics that would enable lossless power transmission, faster MRI machines and superfast levitating trains.
Certain materials involving copper and oxygen display superconductivity (where electricity flows without resistance) at relatively high—but still frigid—temperatures below minus 140 degrees Celsius. At higher temperatures, these materials fall into what’s called the pseudogap state, where they sometimes act like a normal metal and sometimes act more like semiconductors.
Scientists have found that the pseudogap shows up in all so-called high-temperature superconducting materials. But they didn’t understand why or how it shows up, or whether it sticks around as the temperature drops to absolute zero (minus 273.15 degrees Celsius), the unreachable lower limit of temperature at which molecular motion stops.
By better understanding how the pseudogap appears and how it relates to the theoretical properties of the superconductive materials at absolute zero, scientists are getting a clearer picture of those materials, says study co-author Antoine Georges, director of the Flatiron Institute’s Center for Computational Quantum Physics.
“It’s like you have a landscape and a lot of fog, and previously you could just see a few valleys and a few peaks,” he says. “Now the fog is dissipating, and we can see more of the full landscape. It’s really quite an exciting time.”
Quantum physicists can study states such as the pseudogap with computational methods that model the behavior of electrons in a material. But these computations are incredibly difficult because of quantum entanglement, in which electrons become connected and cannot be treated individually even after they separate. For more than a few dozen electrons, directly calculating the behavior of all the particles is impossible.
“Computing the properties of these materials is extraordinarily challenging—you can’t simulate them exactly on even the most powerful computer you can think of,” Georges says. “You have to resort to clever algorithms and simplified models.”
One famous model is called the Hubbard model: Researchers treat the material as a chessboard on which electrons can hop between adjacent spaces like a rook. Electrons can have either an upward or a downward spin. Two electrons can only share a space on the board if they have opposite spins and pay an energy cost. With this model, which originated in the 1960s, scientists can deploy different computational methods, each with strengths and weaknesses in different situations.
“There is a class of methods which work very well at zero temperature, and there is another class of methods which work very well at finite temperatures,” says Fedor Šimkovic IV, lead author of the new study, who was a postdoc with co-author Michel Ferrero at the École Polytechnique and Collège de France in Paris and is now a team lead at IQM Quantum Computers in Munich, Germany. “These two worlds don’t usually speak to each other, because in between them, at very low but finite temperatures, actually lies the computationally hardest regime.”
That in-between state is exactly where the pseudogap lives. To address that regime, the team applied an algorithm called diagrammatic Monte Carlo, which was first described in 1998; it was improved in 2017 by Riccardo Rossi, a co-author of the new paper. Unlike quantum Monte Carlo, a fruitful and well-known algorithm that uses randomness to examine small areas of the model at a time and glues together those examinations to reach conclusions, diagrammatic Monte Carlo considers interactions across the entire chessboard at once.
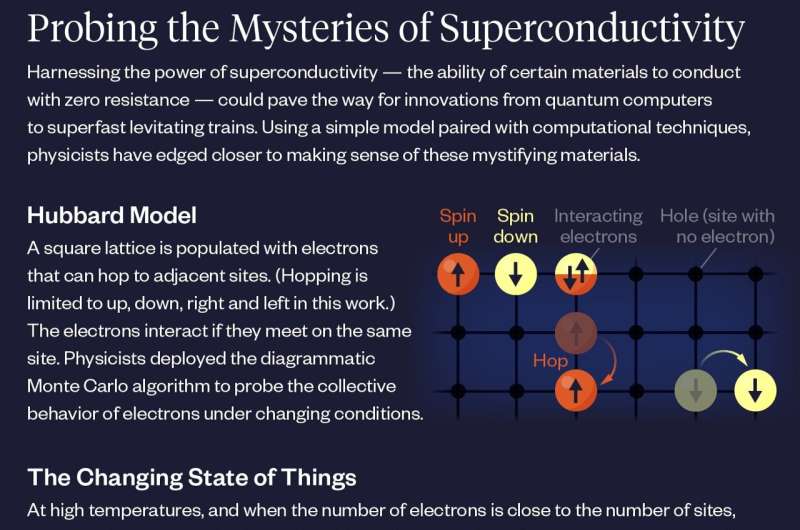
An infographic explaining new research findings about the pseudogap. © Lucy Reading-Ikkanda/Simons Foundation
“The approach of diagrammatic Monte Carlo is very different,” says Rossi, a researcher at CNRS and Sorbonne University. “We can simulate, in principle, an infinite number of particles.”
Armed with diagrammatic Monte Carlo, the team figured out what happens to pseudogap materials as they cool down toward absolute zero. From previous research, they knew that the materials could start superconducting, or they could develop “stripes,” in which the electrons organize into rows of matching spins separated by rows of empty squares.
Which state the Hubbard model enters at absolute zero depends on the number of electrons. When the model includes exactly as many electrons as chessboard squares, the entire board becomes a stable checkerboard pattern of up and down spins, making the material an electric insulator (deeply uninteresting for superconducting research, because insulators are the opposite of conductors). Adding or taking away electrons can cause superconductivity and/or stripes.
At higher temperatures, at which electrons still move around, researchers knew that taking away electrons would cause the pseudogap, but they didn’t know what would happen as the material cooled.
“It was debated if the pseudogap always evolves into the stripe state,” Georges says. “Our paper answers this prominent question in the field and closes that window.” The study revealed that as materials in the pseudogap cool toward absolute zero, they do indeed develop stripes. Interestingly, Georges adds, tweaking the Hubbard model to allow diagonal moves, like a bishop’s, makes the pseudogap evolve into a superconductor as it cools.
The paper also answered the question of what causes the pseudogap, in which the electron arrangement is no longer uniform as it was at absolute zero but instead includes some stripy areas, some squares with two electrons, some holes, and some patches of checkerboard patterns. The researchers figured out that as soon as those checkerboard patches appeared in the electron arrangements, the materials fell into the pseudogap. These two big answers about the pseudogap help further untangle the Hubbard model.
“On a broader level, this whole thing is part of a collective effort across the scientific community of combining together computational approaches to crack open these difficult nuts,” Georges says. “We are living through times where finally these problems are getting clarified.”
These results will also benefit other applications beyond numerical calculations, including quantum gas simulation, a 20-year-old field at the intersection of quantum optics and condensed matter physics. In these experiments, atoms are cooled down to ultracold temperatures and then trapped by lasers into a grid akin to the Hubbard model. With new developments in quantum optics, researchers can now lower those temperatures almost to the point where the pseudogap forms, uniting theory and experiment.
“Our paper has a direct bearing on these ultracold quantum gas simulators,” Georges says. “These quantum simulators are now on the verge of being able to see this pseudogap phenomenon, so I’m expecting some really interesting developments in the coming year or two.”
More information:
Fedor Šimkovic IV, Origin and fate of the pseudogap in the doped Hubbard model, Science (2024). DOI: 10.1126/science.ade9194
Provided by
Simons Foundation
Citation:
Mysteries of the bizarre ‘pseudogap’ in quantum physics finally untangled (2024, September 19)