March 14 is celebrated as Pi Day because the date, when written as 3/14, matches the start of the decimal expansion 3.14159… of the most famous mathematical constant.
By itself, pi is simply a number, one among countless others between 3 and 4. What makes it famous is that it’s built into every circle you see – circumference equals pi times diameter – not to mention a range of other, unrelated contexts in nature, from the bell curve distribution to general relativity.
The true reason to celebrate Pi Day is that mathematics, which is a purely abstract subject, turns out to describe our universe so well. My book “The Big Bang of Numbers” explores how remarkably hardwired into our reality math is. Perhaps the most striking evidence comes from mathematical constants: those rare numbers, including pi, that break out of the pack by appearing so frequently – and often, unexpectedly – in natural phenomena and related equations, that mathematicians like me exalt them with special names and symbols.
So, what other mathematical constants are worth celebrating? Here are my proposals to start filling out the rest of the calendar.
The Golden Ratio
For January, I nominate the Golden Ratio, phi. Two quantities are said to be in this ratio if dividing the larger by the smaller quantity gives the same answer as dividing the sum of the two quantities by the larger quantity. Phi equals 1.618…, and since there’s no Jan. 61, we could celebrate it on Jan. 6.
First calculated by Euclid, this ratio was popularized by Italian mathematician Luca Pacioli, who wrote a book in 1509 extravagantly extolling its aesthetic properties. Supposedly, Leonardo da Vinci, who drew 60 drawings for this book, incorporated it into the dimensions of Mona Lisa’s features, a choice some claim is responsible for her beauty.
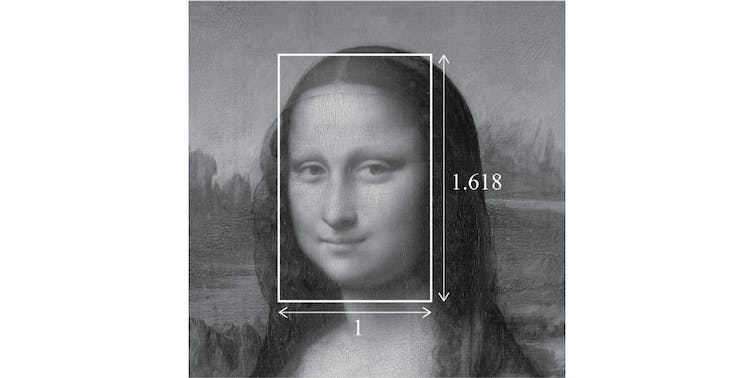
The vertical and horizontal measures of Mona Lisa’s face fit the Golden Ratio.
‘The Big Bang of Numbers’
The first inkling that phi occurs in nature came from another Italian, Fibonacci, while studying how rabbits multiply. A common reproductive assumption was that each pair of rabbits begets another pair every month. Start with a single rabbit pair, and successive populations will then follow the sequence 1, 2, 4, 8, 16, 32, 64, 128, 256 and so on – that is, get multiplied by a monthly “growth ratio” of 2.
What Fibonacci observed, though, was that rabbits spent the first cycle reaching sexual maturity and only began reproducing after that. A single pair now gives the new, slower progression 1, 1, 2, 3, 5, 8, 13, 21, 34… instead. This is the famous sequence named after Fibonacci; notice that each population turns out to be the sum of its two predecessors.
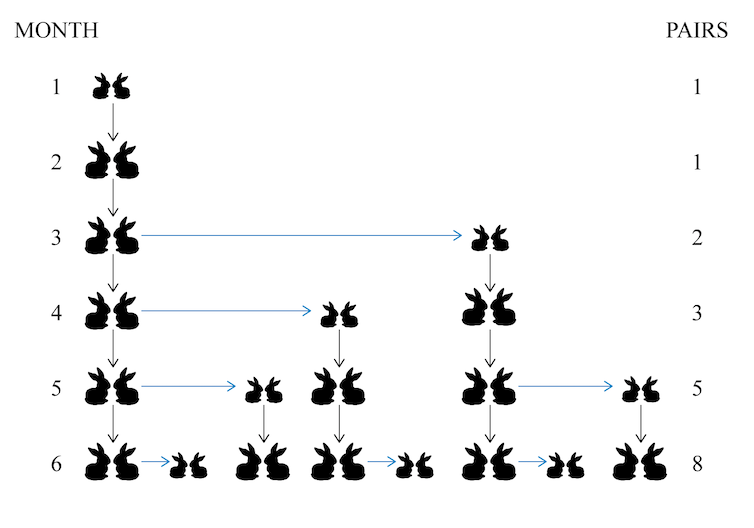
Fibonacci’s rabbits don’t really double their population each generation – their growth ratio actually approaches the 1.618… of phi.
‘The Big Bang of Numbers’
How does phi show up amid…



